



生物多样性 ›› 2011, Vol. 19 ›› Issue (4): 404-413. DOI: 10.3724/SP.J.1003.2011.10020 cstr: 32101.14.SP.J.1003.2011.10020
王宜成
收稿日期:2011-01-26
接受日期:2011-05-25
出版日期:2011-07-20
发布日期:2011-07-29
基金资助:Yicheng Wang
Received:2011-01-26
Accepted:2011-05-25
Online:2011-07-20
Published:2011-07-29
摘要:
生境破碎是导致生物多样性损失的重要原因之一, 在设计自然保护区时设法减少生境破碎是提高保护区有效性的重要方法。由于经济资源或地理因素制约不可能把连续的大片土地都划为保护区时, 设计一个由相互分离的几部分组成的保护区是更为现实的做法。选择地块组成内部间隔最小的保护区是减少破碎化的一个重要途径, 但结合空间特征的保护区地块选择模型求最优解时容易遇到计算困难。Williams(2002)基于原图和对偶图概念, 提出一个线性整数规划模型, 主要用于规划连续的土地。本文对他的模型进行改进, 以确定一个保护一组目标物种、间隔最小的自然保护区。改进后的模型对每个备选地块定义两个变量, 用两个变量的取值差异表示保护区内部间隔。模型的计算效率检验结果显示, 该模型可在合理时间内解决包含100个备选地块的间隔最小保护区设计问题。用美国Illinois州部分地区濒危鸟类保护区设计为例, 设计了多种情形下间隔最小的保护区。线性整数规划模型的计算效率仍需提高, 以解决大型保护区最优化设计问题。模型的实际应用需要完整和准确的数据包括物种分布、选择费用等。
王宜成 (2011) 用原图-对偶图法设计内部间隔最小的自然保护区. 生物多样性, 19, 404-413. DOI: 10.3724/SP.J.1003.2011.10020.
Yicheng Wang (2011) A model for designing nature reserves with minimal fragmentation using a primal-dual graph approach. Biodiversity Science, 19, 404-413. DOI: 10.3724/SP.J.1003.2011.10020.
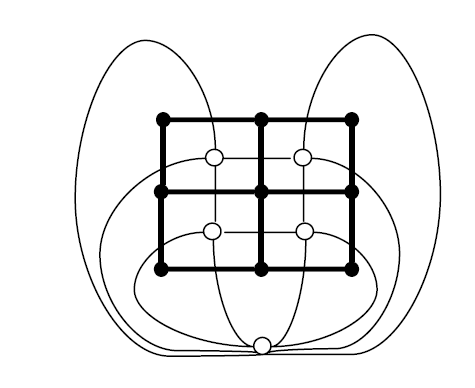
图2 分别包含9个原点和5个对偶点的原图和对偶图以及它们的关系
Fig. 2 Primal and dual graphs consisting of 9 primal nodes and 5 dual nodes, respectively, and their intersecting relation
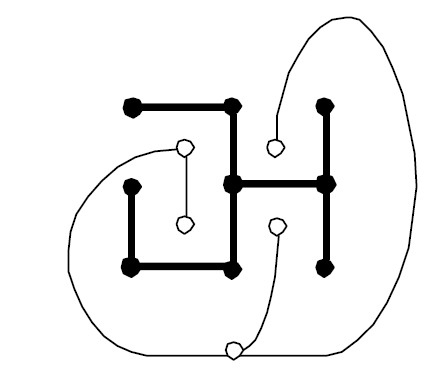
图3 原图和对偶图的互补结构(黑点和粗线组成原图, 圆圈和细线组成对偶图)
Fig. 3 Interwoven structure of primal and dual trees (primal tree consists of black dots and bold lines, and dual tree blank circles and thin lines)
| α | SCP解 SCP solution | r | 模型大小 Model sizea | 目标值 Objective valueb | 时间(秒) Time (s)c | 迭代 Iterationsd | 节点 Nodese |
|---|---|---|---|---|---|---|---|
| 0.8 | 2 | 3 | 0.0 | 3.9 | 11,084 | 61 | |
| 0.7 | 2 | 3 | 0.1 | 10.0 | 34,150 | 238 | |
| 0.6 | 3 | 3 | 952 | 1.6 | 36.2 | 183,910 | 1,810 |
| 0.5 | 3 | 4 | 1,241 | 0.9 | 36.4 | 170,883 | 1,313 |
| 0.4 | 4 | 6 | 200 | 0.1 | 54.6 | 245,866 | 1,308 |
| 0.3 | 5 | 7 | 1.0 | 98.1 | 480,861 | 3,207 | |
| 0.2 | 7 | 9 | 2.8 | 194.0 | 1,056,830 | 8,873 |
表1 间隔最小模型的计算结果(备选地块数量=100, 物种数量=30)
Table 1 Computational results of the minimal fragmentation model (No. of sites = 100, No. of species = 30)
| α | SCP解 SCP solution | r | 模型大小 Model sizea | 目标值 Objective valueb | 时间(秒) Time (s)c | 迭代 Iterationsd | 节点 Nodese |
|---|---|---|---|---|---|---|---|
| 0.8 | 2 | 3 | 0.0 | 3.9 | 11,084 | 61 | |
| 0.7 | 2 | 3 | 0.1 | 10.0 | 34,150 | 238 | |
| 0.6 | 3 | 3 | 952 | 1.6 | 36.2 | 183,910 | 1,810 |
| 0.5 | 3 | 4 | 1,241 | 0.9 | 36.4 | 170,883 | 1,313 |
| 0.4 | 4 | 6 | 200 | 0.1 | 54.6 | 245,866 | 1,308 |
| 0.3 | 5 | 7 | 1.0 | 98.1 | 480,861 | 3,207 | |
| 0.2 | 7 | 9 | 2.8 | 194.0 | 1,056,830 | 8,873 |
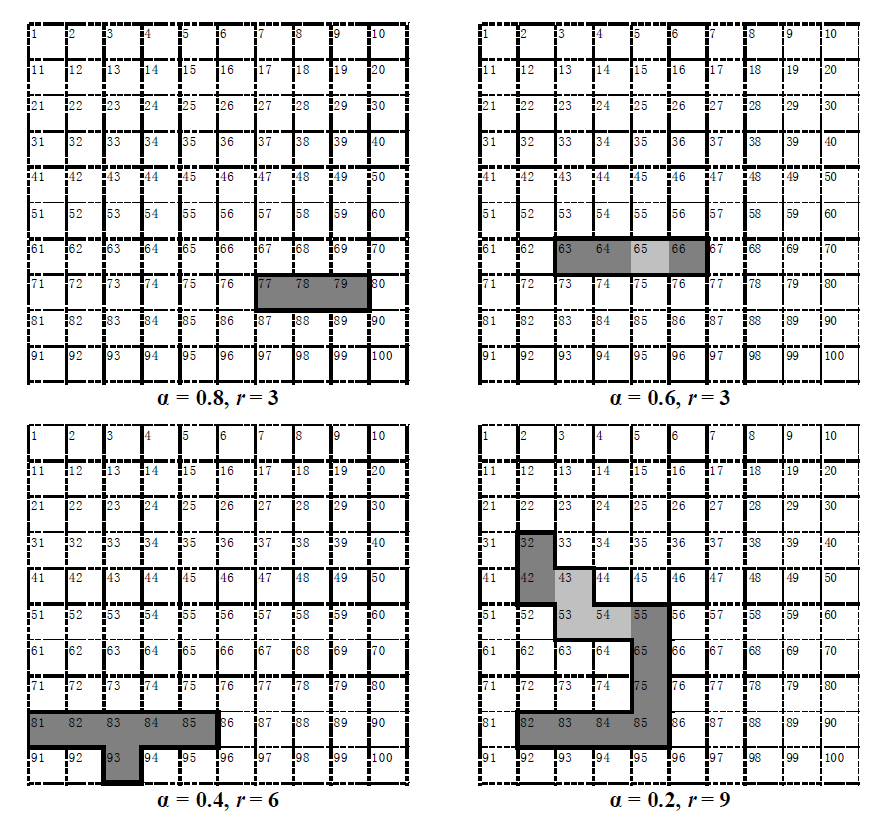
图5 模型的部分运行结果。深灰色地块组成保护区, 浅灰色是间隔。α是可共存于同一地块的物种占物种总数的最大比例, r是选中地块的最大数量。
Fig. 5 Selected solutions of the model. Dark grey cells consist of the reserve, shallow grey cells are fragmentation. α, Ratio of species that can coexist in any potential site to total number of species; r, Maximal number of selected sites.
| [1] | Ando A, Camm JD, Polasky S, Solow A (1998) Species distribution, land values and efficient conservation. Science, 279, 2126-2128. |
| [2] | Bolger DT, Scott TA, Rotenberry JT (2001) Use of corridor-like structures by bird and small mammal species. Biological Conservation, 102, 213-224. |
| [3] | Brooke A, Kendrick D, Meeraus A, Raman R (1998) GAMS― A User’s Guide. GAMS Development Corporation, Washington, DC. |
| [4] | Cabeza M, Araújo MB, Wilson RJ, Thomas CD, Cowley MJR, Moilanen A (2004) Combining probabilities of occurrence with spatial reserve design. Journal of Applied Ecology, 41, 252-262. |
| [5] | Church RL, ReVelle C (1974) The maximal covering location problem. Papers of the Regional Science Association, 32, 101-118. |
| [6] | Church RL, Stoms DM, Davis FW (1996) Reserve selection as a maximal covering location problem. Biological Conservation, 76, 105-112. |
| [7] | Cocks KD, Baird IA (1989) Using mathematical programming to address the multiple reserve selection problem: an example from the Eyre Peninsula, South Australia. Biological Conservation, 78, 113-130. |
| [8] | Costello C, Polasky S (2004) Dynamic reserve site selection. Resource and Energy Economics, 26, 157-174. |
| [9] |
Diestel R (2005) Graph Theory, 3rd edn. Springer, Berlin.
URL PMID |
| [10] | Fischer DT, Church RL (2003) Clustering and compactness in reserve site selection: an extension of the biodiversity management area selection model. Forest Science, 49, 555-565. |
| [11] | Fu W (富伟), Liu SL (刘世梁), Cui BS (崔保山), Zhang ZL (张兆苓) (2009) A review on ecological connectivity in landscape ecology. Acta Ecologica Sinica (生态学报), 29, 6174-6182. (in Chinese with English abstract) |
| [12] | Gao ZX (高增祥), Chen S (陈尚), Li DM (李典谟), Xu RM (徐汝梅) (2007) Origin and essence of island biogeography and metapopulation theory. Acta Ecologica Sinica (生态学报), 27, 304-313. (in Chinese with English abstract) |
| [13] | Haight RG, ReVelle CS, Snyder SA (2000) An integer optimization approach to a probabilistic reserve site selection problem. Operations Research, 48, 697-708. |
| [14] | Haight RG, Travis LE (2008) Reserve design to maximize species persistence. Environmental Modeling and Assessment, 13, 243-253. |
| [15] | Hanski I (1998) Metapopulation dynamics. Nature, 396, 41-49. |
| [16] | Harrison P, Spring D, MacKenzie M, Nally RM (2008) Dynamic reserve design with the union-find algorithm. Ecological Modelling, 215, 369-376. |
| [17] | Hodgson JA, Moilanen A, Wintle BA, Thomas CD (2011) Habitat area, quality and connectivity: striking the balance for efficient conservation. Journal of Applied Ecology, 48, 148-152. |
| [18] | Li XW (李晓文), Hu YM (胡远满), Xiao DN (肖笃宁) (1999) Landscape ecology and biodiversity conservation. Acta Ecologica Sinica (生态学报), 19, 399-407. (in Chinese with English abstract) |
| [19] | Nalle DJ, Arthur JL, Sessions J (2002) Designing compact and contiguous reserve networks with a hybrid heuristic algorithm. Forest Science, 48, 59-68. |
| [20] | Önal H (2003) First-best, second-best, and heuristic solutions in conservation reserve selection. Biological Conservation, 115, 55-62. |
| [21] | Önal H, Briers R (2006) Optimum selection of a connected conservation reserve network. Operations Research, 54, 379-388. |
| [22] | Önal H, Wang Y (2008) A graph theory approach for designing conservation reserve networks with minimal fragmentation. Networks, 52, 142-152. |
| [23] | Possingham H, Ball I, Andelman S (2000) Mathematical methods for identifying representative reserve networks. In: Quantitative Methods for Conservation Biology (eds Ferson S, Burgman M), pp. 291-305. Springer, New York. |
| [24] | Tang ZY (唐志尧), Qiao XJ (乔秀娟), Fang JY (方精云) (2009) Species-area relationship in biological communities. Biodiversity Science (生物多样性), 17, 549-559. (in Chinese with English abstract) |
| [25] | Toregas C, Swain R, ReVelle C, Bergman L (1971) The location of emergency service facilities. Operations Research, 19, 1363-1373. |
| [26] | Underhill LG (1994) Optimal and suboptimal reserve selection algorithms. Biological Conservation, 70, 85-87. |
| [27] | Vanegas P, Cattrysse D, Van Orshoven J (2010) Compactness in spatial decision support: a literature review. Lecture Notes in Computer Science, 6016, 414-429. |
| [28] | Williams JC (2002) A zero-one programming model for contiguous land acquisition. Geographical Analysis, 34, 330-349. |
| [29] | Williams JC, ReVelle CS, Levin SA (2004) Using mathematical optimization models to design nature reserves. Frontiers in Ecology and the Environment, 2, 98-105. |
| [30] | Williams JC, ReVelle CS, Levin SA (2005) Spatial attributes and reserve design models: a review. Environmental Modeling and Assessment, 10, 163-181. |
| [31] | Xu HG (徐海根), Wang LL (王连龙), Bao HS (包浩生) (2003) Designing of nature reserve network: a case study of the red-crowned crane nature reserve. Rural Eco-Environment (农村生态环境), 19(4), 5-9. (in Chinese with English abstract) |
| [1] | 张晶晶, 黄文彬, 陈奕廷, 杨泽鹏, 柯伟业, 彭昭杰, 魏世超, 张志伟, 胡怡思, 余文华, 周文良. 广东南澎列岛海洋生态国家级自然保护区造礁石珊瑚多样性及分布特征[J]. 生物多样性, 2025, 33(4): 24424-. |
| [2] | 王林, 尹梓杨, 黄慧芳, 王静. 基于Carter-Morley Jones蛋形模型的参数估计新方法[J]. 生物多样性, 2025, 33(1): 24203-. |
| [3] | 顾燚芸, 薛嘉祈, 高金会, 谢心仪, 韦铭, 雷进宇, 闻丞. 一种基于公众科学数据的区域性鸟类多样性评价方法[J]. 生物多样性, 2024, 32(7): 24080-. |
| [4] | 李雪萌, 蒋际宝, 张曾鲁, 刘晓静, 王亚利, 吴宜钊, 李银生, 邱江平, 赵琦. 宝天曼国家级自然保护区蚯蚓物种多样性及其影响因素[J]. 生物多样性, 2024, 32(4): 23352-. |
| [5] | 王启蕃, 刘小慧, 朱紫薇, 刘磊, 王鑫雪, 汲旭阳, 周绍春, 张子栋, 董红雨, 张明海. 黑龙江北极村国家级自然保护区鸟类与兽类多样性[J]. 生物多样性, 2024, 32(4): 24024-. |
| [6] | 所翟, 俞渃茜, 李媛辉, 徐基良. 基于实证分析中国自然保护区地方立法问题检视和优化路径[J]. 生物多样性, 2024, 32(2): 23287-. |
| [7] | 刘啸林, 吴友贵, 张敏华, 陈小荣, 朱志成, 陈定云, 董舒, 李步杭, 丁炳扬, 刘宇. 浙江百山祖25 ha亚热带森林动态监测样地群落组成与结构特征[J]. 生物多样性, 2024, 32(2): 23294-. |
| [8] | 黄小龙, 蒙秉顺, 李海波, 冉伟, 杨伟, 王丞, 谢波, 张旭, 冉景丞, 张明明. 基于红外相机的黔金丝猴及其同域分布物种种间关联[J]. 生物多样性, 2024, 32(2): 23402-. |
| [9] | 杨向林, 赵彩云, 李俊生, 种方方, 李文金. 植物入侵导致群落谱系结构更加聚集: 以广西国家级自然保护区草本植物为例[J]. 生物多样性, 2024, 32(11): 24175-. |
| [10] | 毛锐锐, 沈拓, 李慧, 田琳楚, 谭海蓉, 卢李荣, 吴小刚, 范宗骥, 伍国仪, 李杰, 吴勇, 朱弼成, 肖治术. 广东车八岭国家级自然保护区无尾两栖类动物鸣声特征数据集[J]. 生物多样性, 2024, 32(10): 24356-. |
| [11] | 崔国发. 关于自然保护地整合优化工作中几个关键问题的讨论与建议[J]. 生物多样性, 2023, 31(9): 22447-. |
| [12] | 邢超, 林依, 周智强, 赵联军, 蒋仕伟, 林蓁蓁, 徐基良, 詹祥江. 基于DNA条形码技术构建王朗国家级自然保护区陆生脊椎动物遗传资源数据库及物种鉴定[J]. 生物多样性, 2023, 31(7): 22661-. |
| [13] | 陈本平, 陈建武, 凌征文, 杨旭, 陈鑫, 李生强, 杨彪. 四川老君山国家级自然保护区林下鸟兽多样性及动态变化数据集[J]. 生物多样性, 2023, 31(5): 22566-. |
| [14] | 姚雪, 陈星, 戴尊, 宋坤, 邢诗晨, 曹宏彧, 邹璐, 王健. 采集策略对叶附生苔类植物发现概率及物种多样性的重要性[J]. 生物多样性, 2023, 31(4): 22685-. |
| [15] | 赵梦乔, 陈友, 徐正会, 王戌勃, 赵忠良, 徐文川, 何宗辉, 王文华. 云南哀牢山国家级自然保护区东坡垂直带蚂蚁物种多样性[J]. 生物多样性, 2023, 31(11): 23168-. |
| 阅读次数 | ||||||
|
全文 |
|
|||||
|
摘要 |
|
|||||
备案号:京ICP备16067583号-7
Copyright © 2022 版权所有 《生物多样性》编辑部
地址: 北京香山南辛村20号, 邮编:100093
电话: 010-62836137, 62836665 E-mail: biodiversity@ibcas.ac.cn